
I am not sure that angle_to can actually return negative angles, but -0.6 is the same (in radians) as 2*PI-0.6, so you may need to check if it is bigger than PI (or convert it to degrees of course if you like that better: var angle_deg = rad2deg(angle_rad)), you should take a look at the values it returns ( print() for example) and understand them so you can check for them wellģ) you can then rotate the player's direction (movement or velocity?) vector by + or - 90° (which are +-PI/2 radians) The sign (+ or -) of the angle will tell if the enemy is on the left or right side of the player
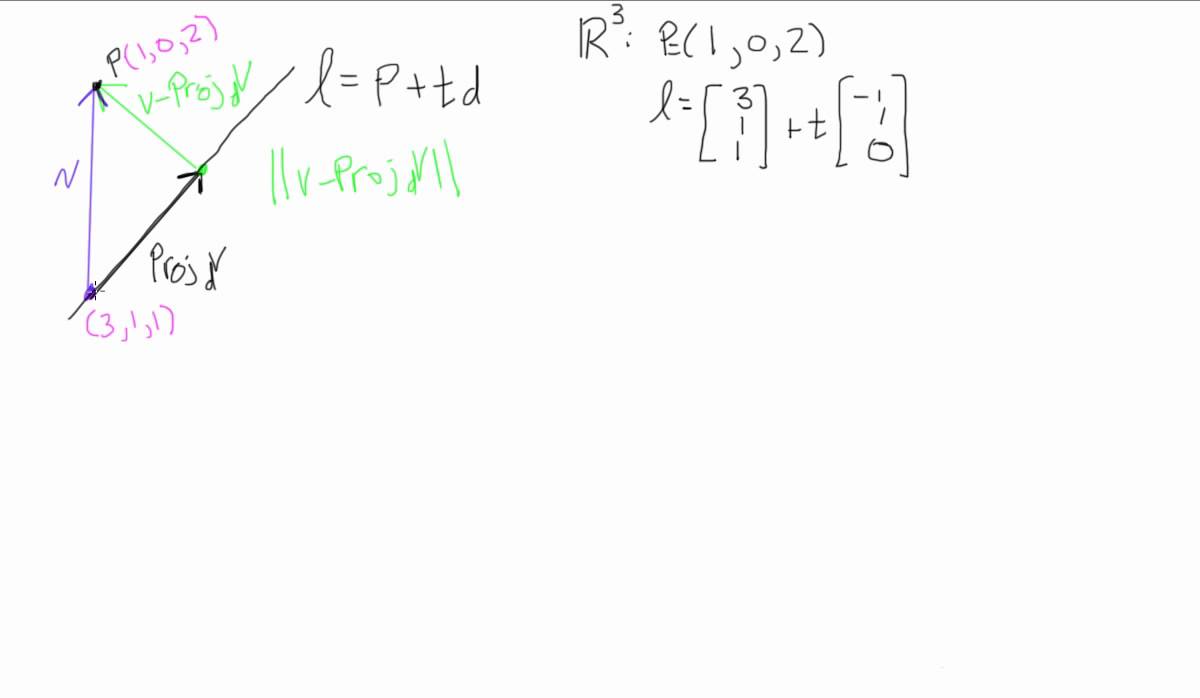
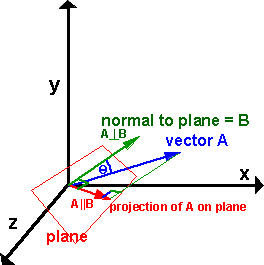
Subtract them like this: var vector_from_player_to_enemy = enemy_position - player_position, there may be a built-in way to do this, the idea is to get the vector that points from the player to the enemyĢ) get the angle from the player's direction to this vector player_direction.angle_to(vector_from_player_to_enemy) How do we define the dot product Dot product (scalar product) of two n-dimensional vectors A and B, is given by this expression. Now the direction numbers of ?a? and ?b? are equal, so we can say that ?a? and ?b? are parallel.So if I needed to do this, I would try doing it this way:ġ) get the enemy's position and the player's position Orthogonal Vectors: Two vectors are orthogonal to each other when their dot product is 0. On the other hand, ?b=\langle-2,4,-6\rangle? has a common factor of ?-2? that can be factored out of the vector. ?a=\langle1,-2,3\rangle? is already irreducible because ?1?, ?-2? and ?3? have no common factors. Since 0 x 0 for any vector x, the zero vector is. Since the dot product is not ?0?, we can say that ?a=\langle1,-2,3\rangle? and ?b=\langle-2,4,-6\rangle? are not orthogonal. Two vectors x, y in R n are orthogonal or perpendicular if x y 0. Therefore, we can say that ?a=2i+3j+5k? and ?b=i+4j-2k? are neither orthogonal nor parallel.įor ?a=\langle1,-2,3\rangle? and ?b=\langle-2,4,-6\rangle?: ?b=\langle1,4,-2\rangle? is also irreducible because ?1?, ?4? and ?-2? have no common factors either. ?a=\langle2,3,5\rangle? is already irreducible because ?2?, ?3? and ?5? have no common factors. To say whether or not the vectors are parallel, we want to look for a common factor in the direction numbers of either vector, and pull it out until both vectors are irreducible.
#Finding orthagonal vector 2d how to
Since the dot product is not ?0?, we can say that ?a=2i+3j+5k? and ?b=i+4j-2k? are not orthogonal. 31,263 views Like Dislike Share Save Christian D 2.49K subscribers In this lesson we cover how to find a vector that is orthogonal (at a right angle) to two other vectors in a three. Let C A ×B Since we know cross product of two vectors produces another vector which is perpendicular to both the vectors. In Python, the row vector and column vector. For example, if you think the answer is vector ñ (1,2,3), you should write n (1, 2, 3) (parentheses make n be a tuple). Tuples are objects with syntax just like an ordered n-tuple in math. Your vector should be defined as a Pythons tuple object named n. Now we’ll take the dot product of our vectors to see whether they’re orthogonal to one another. Question: Question 3 Find a vector ñ which is orthogonal to the plane z 2x - 5y + 3. If we know that they’re orthogonal, then by definition they can’t be parallel, so we’re done with our testing.įor ?a=2i+3j+5k? and ?b=i+4j-2k?:įirst we’ll put the vectors in standard form. Suppose we ask for the first principal component of the residuals. Since the dot product is ?0?, we can say that ?a=\langle2,1\rangle? and ?b=\langle-1,2\rangle? are orthogonal. We know that the residual vectors are all orthogonal to the projections. We’ll take the dot product of our vectors to see whether they’re orthogonal to one another. We begin by finding two points that lie on. Compute properties and norms and do vector algebra computations and projections. Find one vector that is parallel to the line y 3x + 2 and a second vector that is perpendicular to the line. One way to generate the first vector orthogonal to. Get answers to your questions about vectors with interactive calculators. Say whether the following vectors are orthogonal, parallel or neither. Orthogonal means 90 from another vector, and unit vectors have a length of 1. In three-space, three vectors can be mutually perpendicular. Testing three vector pairs to determine whether they are orthogonal, parallel, or neither abcdefg two dimensional motion theory asgarov kochari 20200806 introduction in this experiment we will look at the motion in two dimensions. Two vectors u and v whose dot product is uv0 (i.e., the vectors are perpendicular) are said to be orthogonal.


 0 kommentar(er)
0 kommentar(er)
